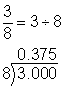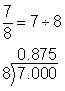Reading: Percents, Decimals, and Fractions
Learning Objective(s)
·Describe the meaning of percent.
·Represent a number as a decimal, percent, and fraction.
Introduction
Three common formats for numbers are fractions, decimals, and percents. Percents are often used to communicate a relative amount. You have probably seen them used for discounts, where the percent of discount can apply to different prices. Percents are also used when discussing taxes and interest rates on savings and loans.
A percent is a ratio of a number to 100. Per cent means "per 100,” or "how many out of 100.” You use the symbol % after a number to indicate percent.
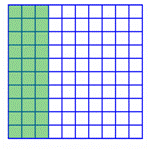
Notice that 12 of the 100 squares in the grid below have been shaded green. This represents 12 percent (12 per 100).
| 12% = 12 percent = 12 parts out of 100 = |
How many of the squares in the grid above are unshaded? Since 12 are shaded and there are a total of 100 squares, 88 are unshaded. The unshaded portion of the whole grid is 88 parts out of 100, or 88% of the grid. Notice that the shaded and unshaded portions together make 100% of the grid (100 out of 100 squares).
Example | |
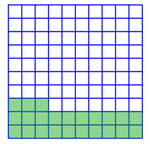
Problem | What percent of the grid is shaded?
|
The grid is divided into 100 smaller squares, with 10 squares in each row. | |
23 squares out of 100 squares are shaded. | |
Answer | 23% of the grid is shaded. |
Example | |
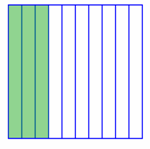
Problem | What percent of the large square is shaded?
|
The grid is divided into 10 rectangles. For percents, you need to look at 100 equal-sized parts of the whole. You can divide each of the 10 rectangles into 10 pieces, giving 100 parts.
| |
30 small squares out of 100 are shaded. | |
Answer | 30% of the large square is shaded. |
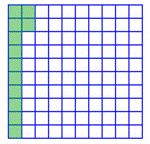
What percent of this grid is shaded?
A) 3% B) 11% C) 38% D) 62% |
It is often helpful to change the format of a number. For example, you may find it easier to add decimals than to add fractions. If you can write the fractions as decimals, you can add them as decimals. Then you can rewrite your decimal sum as a fraction, if necessary.
Percents can be written as fractions and decimals in very few steps.
Example | ||
Problem | Write 25% as a simplified fraction and as a decimal. | |
Write as a fraction. | 25% = | Since % means "out of 100,” 25% means 25 out of 100. You write this as a fraction, using 100 as the denominator. |
| Simplify the fraction by dividing the numerator and denominator by the common factor 25. | |
Write as a decimal. | 25% = | You can also just move the decimal point in the whole number 25 two places to the left to get 0.25. |
Answer | 25% = | |
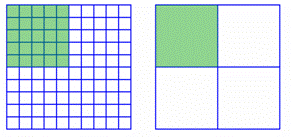
= 0.25Notice in the diagram below that 25% of a grid is also 
of the grid, as you found in the example.
Notice that in the previous example, rewriting a percent as a decimal takes just a shift of the decimal point. You can use fractions to understand why this is the case. Any percentage x can be represented as the fraction 
, and any fraction can be written as a decimal by moving the decimal point in x two places to the left. For example, 81% can be written as 
, and dividing 81 by 100 results in 0.81. People often skip over the intermediary fraction step and just convert a percent to a decimal by moving the decimal point two places to the left.
In the same way, rewriting a decimal as a percent (or as a fraction) requires few steps.
Example | ||
Problem | Write 0.6 as a percent and as a simplified fraction. | |
Write as a percent. | 0.6 = 0.60 = 60% | Write 0.6 as 0.60, which is 60 hundredths. 60 hundredths is 60 percent. You can also move the decimal point two places to the right to find the percent equivalent. |
Write as a fraction. | 0.6 = | To write 0.6 as a fraction, you read the decimal, 6 tenths, and write 6 tenths in fraction form. |
| Simplify the fraction by dividing the numerator and denominator by 2, a common factor. | |
Answer | 0.6 = 60% = | |
In this example, the percent is not a whole number. You can handle this in the same way, but it's usually easier to convert the percent to a decimal and then convert the decimal to a fraction.
Example | |||
Problem | Write 5.6% as a decimal and as a simplified fraction. | ||
Write as a decimal. | 5.6% = 0.056 | Move the decimal point two places to the left. In this case, insert a 0 in front of the 5 (05.6) in order to be able to move the decimal to the left two places. | |
Write as a fraction. | 0.056 = | Write the fraction as you would read the decimal. The last digit is in the thousandths place, so the denominator is 1,000. | |
| Simplify the fraction by dividing the numerator and denominator by 8, a common factor. | ||
Answer | 5.6% = | ||
= 0.056Write 0.645 as a percent and as a simplified fraction. A) 64.5% and B) 0.645% and C) 645% and D) 64.5% and |
In order to write a fraction as a decimal or a percent, you can write the fraction as an equivalent fraction with a denominator of 10 (or any other power of 10 such as 100 or 1,000), which can be then converted to a decimal and then a percent.
Example | ||
Problem | Write | |
Write as a decimal. |
| Find an equivalent fraction with 10, 100, 1,000, or other power of 10 in the denominator. Since 100 is a multiple of 4, you can multiply 4 by 25 to get 100. Multiply both the numerator and the denominator by 25. |
| Write the fraction as a decimal with the 5 in the hundredths place. | |
Write as a percent. | 0.75 = 75% | To write the decimal as a percent, move the decimal point two places to the right. |
Answer |
| |
as a decimal and as a percent.
= 0.75
If it is difficult to find an equivalent fraction with a denominator of 10, 100, 1,000, and so on, you can always divide the numerator by the denominator to find the decimal equivalent.
Example | ||
Problem | Write | |
Write as a decimal. |
| Divide the numerator by the denominator. 3 ÷ 8 = 0.375. |
Write as a percent. | 0.375 = 37.5% | To write the decimal as a percent, move the decimal point two places to the right. |
Answer |
| |
as a decimal and as a percent.
= 0.375 = 37.5%Write A) 80.0 and 0.8% B) 0.4 and 4% C) 0.8 and 80% D) 0.8 and 8% |
as a decimal and as a percent.Mixed Numbers
All the previous examples involve fractions and decimals less than 1, so all of the percents you have seen so far have been less than 100%.
Percents greater than 100% are possible as well. Percents more than 100% are used to describe situations where there is more than one whole (fractions and decimals greater than 1 are used for the same reason).
In the diagram below, 115% is shaded. Each grid is considered a whole, and you need two grids for 115%.
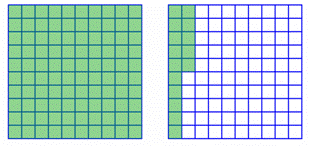
Expressed as a decimal, the percent 115% is 1.15; as a fraction, it is 
, or 
. Notice that you can still convert among percents, fractions, and decimals when the quantity is greater than one whole.
Numbers greater than one that include a fractional part can be written as the sum of a whole number and the fractional part. For instance, the mixed number 
is the sum of the whole number 3 and the fraction . = 3 + .
Example | ||
Problem | Write | |
| Write the mixed fraction as 2 wholes plus the fractional part. | |
Write as a decimal. |
| Write the fractional part as a decimal by dividing the numerator by the denominator. 7 ÷ 8 = 0.875. |
| Add 2 to the decimal. | |
Write as a percent. | 2.875 = 287.5% | Now you can move the decimal point two places to the right to write the decimal as a percent. |
Answer |
| |
as a decimal and as a percent.
= 2.875 = 287.5%Note that a whole number can be written as a percent. 100% means one whole; so two wholes would be 200%.
Example | ||
Problem | Write 375% as a decimal and as a simplified fraction. | |
Write as a decimal. | 375% = 3.75 | Move the decimal point two places to the left. Note that there is a whole number along with the decimal as the percent is more than 100%. |
Write as a fraction. | 3.75 = 3 + 0.75 | Write the decimal as a sum of the whole number and the fractional part. |
0.75 = | Write the decimal part as a fraction. | |
| Simplify the fraction by dividing the numerator and denominator by a common factor of 25. | |
3 + | Add the whole number part to the fraction. | |
Answer | 375% = 3.75= | |
= 
Write 4.12 as a percent and as a simplified fraction. A) 0.0412% and B) 412% and C) 412% and D) 4.12% and |
Summary
Percents are a common way to represent fractional amounts, just as decimals and fractions are. Any number that can be written as a decimal, fraction, or percent can also be written using the other two representations.
Permissions
This reading is taken from the Developmental Math Open Program created by The NROC Project. It is available under a Creative Commons license.
Последнее изменение: четверг, 25 августа 2016, 10:00