Reading: Multiplying and Dividing with Decimals
Learning Objective(s)
·Multiply two or more decimals.
·Multiply a decimal by a power of 10.
·Divide by a decimal.
·Divide a decimal by a power of 10.
·Solve application problems that require decimal multiplication or division.
Introduction
As with whole numbers, sometimes you run into situations where you need to multiply or divide decimals. And just as there is a correct way to multiply and divide whole numbers, so, too, there is a correct way to multiply and divide decimals.
Imagine that a couple eats dinner at a Japanese steakhouse. The bill for the meal is $58.32--which includes a tax of $4.64. To calculate the tip, they can double the tax. So if they know how to multiply $4.64 by 2, the couple can figure out how much they should leave for the tip.
Here's another problem. Andy just sold his van that averaged 20 miles per gallon of gasoline. He bought a new pickup truck and took it on a trip of 614.25 miles. He used 31.5 gallons of gas to make it that far. Did Andy get better gas mileage with the new truck?
Both of these problems can be solved by multiplying or dividing decimals. Here's how to do it.
Multiplying decimals is the same as multiplying whole numbers except for the placement of the decimal point in the answer. When you multiply decimals, the decimal point is placed in the product so that the number of decimal places in the product is the sum of the decimal places in the factors.
Let's compare two multiplication problems that look similar: 214 · 36, and 21.4 · 3.6.
214 x 36 1284 6420 7,704 | 21.4 x 3.6 1284 6420 77.04 |
Notice how the digits in the two solutions are exactly the same - the multiplication does not change at all. The difference lies in the placement of the decimal point in the final answers: 214 · 36 = 7,704, and 21.4 · 3.6 = 77.04.
To find out where to put the decimal point in a decimal multiplication problem, count the total number of decimal places in each of the factors.
21.4 the first factor has one decimal place
3.6 the second factor has one decimal place
77.04 the product will have 1 + 1 = 2 decimal places
Note that the decimal points do not have to be aligned as for addition and subtraction.
Example | |||
Problem | 3.04 · 6.1 = ? | ||
3.04 x 6.1 304 18240 18544 | Set up the problem. Multiply. Add. | ||
3.04 x 6.1 304 18240 18.544 | ← ← ← | Count the total number of decimal places in the factors and insert the decimal point in the product. 2 decimal places. 1 decimal place. 3 decimal places. | |
Answer | 3.04 · 6.1 = 18.544 | ||
Sometimes you may need to insert zeros in front of the product so that you have the right number of decimal places. See the final answer in the example below:
Example | |||
Problem | 0.037 · 0.08 = ? | ||
0.037 x 0.08 296 | Set up the problem. Multiply. | ||
0.037 x 0.08 0.00296 | ← ← ← | Count the total number of decimal places in the factors and insert the decimal point in the product. 3 decimal places. 2 decimal places. 5 decimal places. | |
Answer | 0.037 · 0.08 = 0.00296 | Note that you needed to add zeros before 296 to get the 5 decimal places. | |
If one or more zeros occur on the right in the product, they are not dropped until after the decimal point is inserted.
Example | |||
Problem | 2.04 · 1.95 = ? | ||
2.04 x 1.95 1020 18360 20400 39780 | Set up the problem. Multiply. Add. | ||
2.04 x 1.95 1020 18360 20400 3.9780 | ← ← ← | 2 decimal places. 2 decimal places. 4 decimal places. | |
Answer | 2.04 · 1.95 = 3.978 | Answer can omit the final trailing 0. | |
Multiplying Decimals To multiply decimals: ·Set up and multiply the numbers as you do with whole numbers. ·Count the total number of decimal places in both of the factors. ·Place the decimal point in the product so that the number of decimal places in the product is the sum of the decimal places in the factors. ·Keep all zeros in the product when you place the decimal point. You can drop the zeros on the right once the decimal point has been placed in the product. If the number of decimal places is greater than the number of digits in the product, you can insert zeros in front of the product. |
Multiply. 51.2 · 3.08 A) 15769.6 B) 1576.96 C) 157.696 D) 15.7696 |
Take a moment to multiply 4.469 by 10. Now do 4.469 · 100. Finally, do 4.469 · 1,000. Notice any patterns in your products?
4.469 x 10 44.690 | 4.469 x 100 446.900 | 4.469 x 1000 4469.000 |
Notice that the products keep getting greater by one place value as the multiplier (10, 100, and 1,000) increases. In fact, the decimal point moves to the right by the same number of zeros in the power of ten multiplier.
4.469 · 10 = 44.69 ^ | 4.469 · 100 = 446.9 ^ | 4.469 · 1,000 = 4469. ^ |
You can use this observation to help you quickly multiply any decimal by a power of ten (10, 100, 1,000, etc).
Example | |||
Problem | 0.03 · 100 = ? | ||
0.03 · 100 = ? | 100 has two zeros. | ||
0.03 · 100 = 3 | Move the decimal point two places to the right to find the product. | ||
Answer | 0.03 · 100 = 3 | ||
Multiplying a Decimal by a Power of Ten To multiply a decimal number by a power of ten (such as 10, 100, 1,000, etc.), count the number of zeros in the power of ten. Then move the decimal point that number of places to the right. For example, 0.054 · 100 = 5.4. The multiplier 100 has two zeros, so you move the decimal point in 0.054 two places to the right--for a product of 5.4. |
To divide decimals, you will once again apply the methods you use for dividing whole numbers. Look at the two problems below. How are the methods similar?
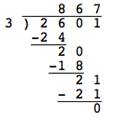
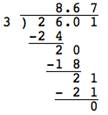
Notice that the division occurs in the same way--the only difference is the placement of the decimal point in the quotient.
Example | |||
Problem | 18.32 ÷ 8 = ? | ||
________ 8 ) 1 8.3 2 | Set up the problem. | ||
2.2 9 8 ) 1 8.3 2 -1 6 2 3 -1 6 7 2 - 7 2 0 | Divide. | ||
2.2 9 8 ) 1 8.3 2 | Place decimal point in the quotient. It should be placed directly above the decimal point in the dividend. | ||
Answer | 18.32 ÷ 8 = 2.29 | ||
But what about a case where you are dividing by a decimal, as in the problem below?
__________ 0.3 ) 2 6 0.1 |
In cases like this, you can use powers of 10 to help create an easier problem to solve. In this case, you can multiply the divisor, 0.3, by 10 to move the decimal point 1 place to the right. If you multiply the divisor by 10, then you also have to multiply the dividend by 10 to keep the quotient the same. The new problem, with its solution, is shown below.
Example | |||
Problem | 260.1 ÷ 0.3 = ? | ||
________ 0.3 ) 2 6 0.1 | Set up the problem. | ||
__________ 3. ) 2 6 0 1. | Multiply divisor and dividend by 10 to create a whole number divisor. | ||
8 6 7 3 ) 2 6 0 1 -2 4 2 0 -1 8 2 1 - 2 1 0 | Divide. | ||
Answer | 260.1 ÷ 0.3 = 867 | ||
Often, the dividend will still be a decimal after multiplying by a power of 10. In this case, the placement of the decimal point must align with the decimal point in the dividend.
Example | |||
Problem | 15.275 ÷ 3.25 = ? | ||
__________ 3.2 5 ) 1 5.2 7 5 | Set up the problem. | ||
___________ 3 2 5.) 1 5 2 7.5 | Multiply divisor and dividend by 100 to create a whole number divisor. | ||
4.7 3 2 5.) 1 5 2 7.5 -1 3 0 0 2 2 7 5 -2 2 7 5 0 | Divide. 325 goes into 1527 four times, so the number 4 is placed above the digit 7. The decimal point in the quotient is placed directly above the decimal point in the dividend. | ||
Answer | 15.275 ÷ 3.25 = 4.7 | ||
Dividing Decimals Dividing Decimals by Whole NumbersDivide as you would with whole numbers. Then place the decimal point in the quotient directly above the decimal point in the dividend. Dividing by DecimalsTo divide by a decimal, multiply the divisor by a power of ten to make the divisor a whole number. Then multiply the dividend by the same power of ten. You can think of this as moving the decimal point in the dividend the same number of places to the right as you move the decimal point in the divisor. Then place the decimal point in the quotient directly over the decimal point in the dividend. Finally, divide as you would with whole numbers. |
Divide: 25.095 ÷ 0.5. A) 5,019 B) 501.9 C) 50.19 D) 0.5019 |
Recall that when you multiply a decimal by a power of ten (10, 100, 1,000, etc), the placement of the decimal point in the product will move to the right according to the number of zeros in the power of ten. For instance, 4.12 · 10 = 41.2.
Multiplication and division are inverse operations, so you can expect that if you divide a decimal by a power of ten, the decimal point in the quotient will also correspond to the number of zeros in the power of ten. The difference is that the decimal point moves to the right when you multiply; it moves to the left when you divide.
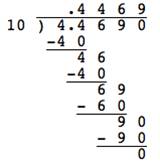
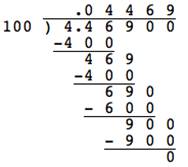
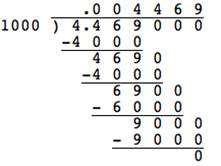
In the examples above, notice that each quotient still contains the digits 4469--but as another 0 is added to the end of each power of ten in the divisor, the decimal point moves an additional place to the left in the quotient.
Dividing by Powers of Ten To divide a decimal by a power of ten (10, 100, 1,000, etc.), count the number of zeros in the divisor. Then move the decimal point in the dividend that number of decimal places to the left; this will be your quotient. |
Example | |||
Problem | 31.05 ÷ 10 = ? | ||
31.05 ÷ 10 = ? | 10 has one zero. | ||
31.05 ÷ 10 = 3.105 | Move the decimal point one place to the left in the dividend; this is the quotient. | ||
Answer | 31.05 ÷ 10 = 3.105 | ||
Divide. 0.045 ÷ 100 A) 0.00045 B) 0.045 C) 4.5 D) 4,500 |
Now let's return to the two problems from the beginning of this section. You know how to multiply and divide with decimals now. Let's put that knowledge to the test.
Example | |||
Problem | A couple eats dinner at a Japanese steakhouse. The bill for the meal totals $58.32--which includes a tax of $4.64. To calculate the tip, they can double the tax. How much tip should the couple leave? | ||
4.64 x 2 | Set up a multiplication problem. | ||
4.64 x 2 928 | Multiply. | ||
4.64 x 2 9.28 | Count the number of decimal places in the two factors, and place the decimal point accordingly. | ||
Answer | The couple should leave a tip of $9.28. | ||
Example | |||
Problem | Andy just sold his van that averaged 20 miles per gallon of gasoline. He bought a new pickup truck and took it on a trip of 614.25 miles. He used 31.5 gallons of gas for the trip. Did Andy get better gas mileage with the new truck? | ||
__________ 3 1.5 ) 6 1 4.2 5 | Set up a division problem. | ||
__________ 3 1 5.) 6 1 4 2.5 | Make the divisor a whole number by multiplying by 10; do the same to the dividend. | ||
1 9.5 3 1 5.) 6 1 4 2.5 -3 1 5 2 9 9 2 -2 8 3 5 1 5 7 5 -1 5 7 5 0 | Divide. Insert a decimal point in the quotient so that it is directly above the decimal point in the dividend. | ||
Answer | Andy gets 19.5 miles per gallon now. He used to get 20 miles per gallon. He does not get better gas mileage with the new truck. | ||
Summary
Learning to multiply and divide with decimals is an important skill. In both cases, you work with the decimals as you have worked with whole numbers, but you have to figure out where the decimal point goes. When multiplying decimals, the number of decimal places in the product is the sum of the decimal places in the factors. When dividing by decimals, move the decimal point in the dividend the same number of places to the right as you move the decimal point in the divisor. Then place the decimal point in the quotient above the decimal point in the dividend.
Permissions
This reading is taken from the Developmental Math Open Program created by The NROC Project. It is available under a Creative Commons license.
