For further reading: The Pythagoreans

Please note: this material is not included in the content for the quiz.  I offer it to you as a way to deepen your understanding of an ancient group of philosophers.  This material was retrieved from     https://en.wikipedia.org/wiki/Pythagoreanism  All the footnote numbers can be examined on the Wikipedia site     --Bob Zomermaand

Philosophy

Early Pythagoreanism was based on research and the accumulation of knowledge from the books written by other philosophers.[7] Pythagoras' philosophic teachings made direct reference to the philosophy of Anaximander, Anaximenes of Miletus and Pherecydes of Syros.[7] Of the Pythagorean philosophers Hippasus, Alcmaeon, Hippon, Archytas and Theodorus written sources have survived.[31]

Arithmetic and numbers

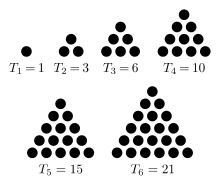
The first six triangular numbers

Pythagoras in his teachings cultivated mathematics and numbers, engaging in a combination of philosophic theorizing and deductive provable methodology. Numbers were in the Greek world of Pythagoras' days natural numbers - that is positive integers. But unlike their Greek contemporaries, the Pythagorean philosophers represented numbers graphically, not symbolically through letters. Pythagoreans used dots, also known as psiphi (pebbles), to represent numbers in triangles, squares, rectangles and pentagons. This enabled a visual comprehension of mathematics and allowed for a geometrical exploration of numerical relationships. Pythagorean philosophers investigated the relationship of numbers exhaustively. They defined perfect numbers as those that were equal the sum of all their divisors. For example: 28 = 1 + 2 + 4 + 7 + 14.[32] The theory of odd and even numbers was central to Pythagorean arithmetic. This distinction was for the Pythagorean philosophers direct and visual, as they arranged triangular dots so that the even and odd numbers successively alternate: 2, 4, 6, ... 3, 5, 7, ...[33]

Early-Pythagorean philosophers such as Philolaus and Archytas held the conviction that mathematics could help in addressing important philosophical problems.[34] In Pythagoreanism numbers became related to intangible concepts. The one was related to the intellect and being, the two to thought, the number four was related to justice because 2 * 2 = 4 and equally even. A dominant symbolism was awarded to the number three, Pythagoreans believed that the whole world and all things in it are summed up in this number, because end, middle and beginning give the number of the whole. The triad had for Pythagoreans an ethical dimension, as the goodness of each person was believed to be threefold - prudence, drive and good fortune.[35]

Geometry

The Pythagoreans engaged with geometry as a liberal philosophy which served to establish principles and allowed theorems to be explored abstractly and mentally. Pythagorean philosophers believed that there was a close relationship between numbers and geometrical forms. Early-Pythagorean philosophers proved simple geometrical theorems, including "the sum of the angles of a triangle equals two right angles". Pythagoreans also came up with three of the five regular polyhedra: the tetrahedron, the cube and the dodecahedron. The sides of a regular dodecahedron are regular pentagons, which for Pythagoreans symbolized health. They also revered the pentagram, as each diagonal divides the two others at the golden ratio.[33] When linear geometrical figures replaced the dots, the combination of Babylonian algebra and Pythagorean arithmetic provided the basis for Greek geometric algebra. By attempting to establish a system of concrete and permanent rules, Pythagoreans helped to establish strict axiomatic procedures of solving mathematical problems.[36]

Music

Image

Medieval woodcut by Franchino Gaffurio, depicting Pythagoras and Philolausconducting musical investigations.

Pythagoras pioneered the mathematical and experimental study of music. He objectively measured physical quantities, such as the length of a string and discovered quantitative mathematical relationships of music through arithmetic ratios. Pythagoras attempted to explain subjective psychological and aesthetic feelings, such as the enjoyment of musical harmony. Pythagoras and his students experimented systematically with strings of varying length and tension, with wind instruments with brass discs of the same diameter but different thicknesses, and with identical vases filled with different levels of water. Early Pythagoreans established quantitative ratios between the length of a string or pipe and the pitch of notes and the frequency of string vibration.[36]

Pythagoras is credited with discovering that the most harmonious musical intervals are created by the simple numerical ratio of the first four integer numbers which derive respectively from the relations of string length: the eighth (1/2), the fifth (2/3) and the fourth (3/4).[36] The sum of those numbers 1 + 2 + 3 + 4 = 10 was for Pythagoreans the perfect number, because it contained in itself "the whole essential nature of numbers". Werner Heisenberg has called this formulation of musical arithmetical as "among the most powerful advances of human science" because it enables the measurement of sound in space.[37]

Pythagorean tuning is a system of musical tuning in which the frequency ratios of all intervals are based on the ratio 3:2.[38]This ratio, also known as the "pure" perfect fifth, is chosen because it is one of the most consonant and easiest to tune by ear and because of importance attributed to the integer 3. As Novalis put it, "The musical proportions seem to me to be particularly correct natural proportions."[39]

The fact that mathematics could explain the human sentimental world had a profound impact on the Pythagorean philosophy. Pythagoreanism became the quest for establishing the fundamental essences of reality. Pythagorean philosophers advanced the unshakable belief that the essence of all thing are numbers and that the universe was sustained by harmony.[37] According to ancient sources music was central to the lives of those practicing Pythagoreanism. They used medicines for the purification (katharsis) of the body and, according to Aristoxenus, music for the purification of the soul. Pythagoreans used different types of music to arouse or calm their souls.[40]

Harmony

For Pythagoreans harmony signified the "unification of a multifarious composition and the agreement of unlike spirits". In Pythagoreanism numeric harmony was applied in mathematical, medical, psychological, aesthetic, metaphysical and cosmological problems. For Pythagorean philosophers the basic property of numbers was expressed in the harmonious interplay of opposite pairs. Harmony assured the balance of opposite forces.[41] Pythagoras had in his teachings named numbers and the symmetries of them as the first principle, and called these numeric symmetries harmony.[42] This numeric harmony could be discovered in rules throughout nature. Numbers governed the properties and conditions of all beings and were regarded the causes of being in everything else. Pythagorean philosophers believed that numbers were the elements of all beings and the universe as a whole was composed of harmony and numbers.[35]

Cosmology

According to a collection of ancient philosophical texts by Stobaeus in the 5th century AD, Philolaus believed there was a "Counter-Earth" (Antichthon) orbiting a "central fire" but not visible from Earth.[43]

The philosopher Philolaus, one of the most prominent figures in Pythagoreanism,[44] was the precursor of Copernicus in moving the earth from the center of the cosmos and making it a planet.[44] According to Aristotle's student Eudemus of Cyprus, the first philosopher to determine quantitatively the size of the known planets and the distance between them was Anaximander, a teacher to Pythagoras, in the 6th century BC. Historic sources credit the Pythagorean philosophers with being the first to attempt a clarification of the planet sequence.[45] The early-Pythagorean philosopher Philolaus believed that limited and unlimited things were the components of the cosmos and these had existed ever since. The center of the universe, according to Philolaus, was the number one (hēn), which equated to the unity of Monism. Philolaus called the number one an "even-odd" because it was able to generate both even and odd numbers. When one was added to an odd number it produced an even number, and when added to an even number it produced an odd number. Philolaus further reasoned that the fitting together of the earth and the universe corresponded to the construction of the number one out of the even and the odd. Pythagorean philosophers believed that the even was unlimited and the odd was limited.[46]