Internal Rate of Return (IRR)

•Internal rate of return (IRR) is the discount rate at which the net present value of an investment becomes zero. In other words, IRR is the discount rate which equates the present value of the future cash flows of an investment with the initial investment. It is one of the several measures used for investment appraisal.
•
•Decision Rule
- A project should only be accepted if its IRR is NOT less than the target internal rate of return. When comparing two or more mutually exclusive projects, the project having highest value of IRR should be accepted.


IRR Calculation

•The calculation of IRR is a bit complex than other capital budgeting techniques. We know that at IRR, Net Present Value (NPV) is zero, thus:

-NPV = 0; or
- PV of future cash flows − Initial Investment = 0; or




IRR Problem

•But the problem is, we cannot isolate the variable r (=internal rate of return) on one side of the above equation. However, there are alternative procedures which can be followed to find IRR. The simplest of them is described below:
•
1.Guess the value of r and calculate the NPV of the project at that value.
2.If NPV is close to zero then IRR is equal to r.
3.If NPV is greater than 0 then increase r and jump to step 5.
4.If NPV is smaller than 0 then decrease r and jump to step 5.
5.Recalculate NPV using the new value of r and go back to step 2.


IRR Example

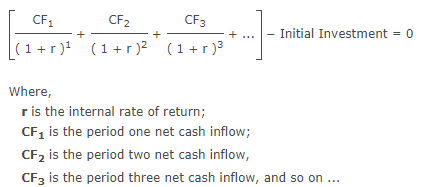
Find the IRR of an investment having initial cash outflow of $213,000. The cash inflows during the first, second, third and fourth years are expected to be $65,200, $96,000, $73,100 and $55,400 respectively.

Solution

Assume that r is 10%.

NPV at 10% discount rate = $18,372

Since NPV is greater than zero we have to increase discount rate, thus

NPV at 13% discount rate = $4,521

But it is still greater than zero we have to further increase the discount rate, thus

NPV at 14% discount rate = $204

NPV at 15% discount rate = ($3,975)

Since NPV is fairly close to zero at 14% value of r, therefore

IRR ≈ 14%


Payback Period

•Payback period is the time in which the initial cash outflow of an investment is expected to be recovered from the cash inflows generated by the investment. It is one of the simplest investment appraisal techniques.
•Decision Rule
- Accept the project only if its payback period is LESS than the target payback period.


Payback Period Formula

The formula to calculate payback period of a project depends on whether the cash flow per period from the project is even or uneven. In case they are even, the formula to calculate payback period is:


When cash inflows are uneven, we need to calculate the cumulative net cash flow for each period and then use the following formula for payback period:


Payback Period Example

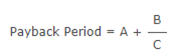
Example 1: Even Cash Flows

Company C is planning to undertake a project requiring initial investment of $105 million. The project is expected to generate $25 million per year for 7 years. Calculate the payback period of the project.

Solution
Payback Period = Initial Investment ÷ Annual Cash Flow = $105M ÷ $25M = 4.2 years


Payback Period Example


Example 2: Uneven Cash Flows

Company C is planning to undertake another project requiring initial investment of $50 million and is expected to generate $10 million in Year 1, $13 million in Year 2, $16 million in year 3, $19 million in Year 4 and $22 million in Year 5. Calculate the payback value of the project.




Payback Period
= 3 + (|-$11M| ÷ $19M)
= 3 + ($11M ÷ $19M)
≈ 3 + 0.58
≈ 3.58 years


Последнее изменение: вторник, 14 августа 2018, 08:51